In this Nrich problem students build on their existing knowledge of proportions to build a function in an engaging context.

This first course in the physics curriculum introduces classical mechanics. Historically, a set of core concepts—space, time, mass, force, momentum, torque, and angular momentum—were introduced in classical mechanics in order to solve the most famous physics problem, the motion of the planets.

" We will study the fundamental principles of classical mechanics, with a modern emphasis on the qualitative structure of phase space. We will use computational ideas to formulate the principles of mechanics precisely. Expression in a computational framework encourages clear thinking and active exploration. We will consider the following topics: the Lagrangian formulation; action, variational principles, and equations of motion; Hamilton's principle; conserved quantities; rigid bodies and tops; Hamiltonian formulation and canonical equations; surfaces of section; chaos; canonical transformations and generating functions; Liouville's theorem and PoincarĚŠ integral invariants; PoincarĚŠ-Birkhoff and KAM theorems; invariant curves and cantori; nonlinear resonances; resonance overlap and transition to chaos; properties of chaotic motion. Ideas will be illustrated and supported with physical examples. We will make extensive use of computing to capture methods, for simulation, and for symbolic analysis."

Students learn about gear ratios and power by operating toy mechanical cranes of differing gear ratios. They attempt to pick up objects with various masses to witness how much power must be applied to the system to oppose the force of gravity. They learn about the concept of gear ratio and practice calculating gear ratios on worksheets, discovering that smaller gear ratios are best for picking objects up quickly, and larger gear ratios make it easier to lift heavy objects.

Investigate collisions on an air hockey table. Set up your own experiments: vary the number of discs, masses and initial conditions. Is momentum conserved? Is kinetic energy conserved? Vary the elasticity and see what happens.

Watch your solution change color as you mix chemicals with water. Then check molarity with the concentration meter. What are all the ways you can change the concentration of your solution? Switch solutes to compare different chemicals and find out how concentrated you can go before you hit saturation!

This is a mathematical number sense puzzle designed for partners to work through.

This short video and interactive assessment activity is designed to give fifth graders an overview of comparing decimals.

This Nrich problem is intentionally difficult as it stands, but this allows us to focus on promoting resilience and perseverance with children.

In this engineering, math, and sustainability project students answer the question, “Can I ride 53 miles on a bike from the energy of a single burrito?” They must define their variables, collect and analyze their data, and present their results. By the end of this project, developed by Allen Distinguished Educator Mike Wierusz, students should have all the information they need to design a burrito that would provide them with the exact caloric content necessary to ride 53 miles.

In this first part of a two-part lab activity, students use triple balance beams and graduated cylinders to take measurements and calculate the densities of several common, irregularly shaped objects with the purpose to resolve confusion about mass and density. After this activity, conduct the associated Density Column Lab - Part 2 activity before presenting the associated Density & Miscibility lesson for discussion about concepts that explain what students have observed.

Concluding a two-part lab activity, students use triple balance beams and graduated cylinders to take measurements and calculate densities of several household liquids and compare them to the densities of irregularly shaped objects (as determined in Part 1). Then they create density columns with the three liquids and four solid items to test their calculations and predictions of the different densities. Once their density columns are complete, students determine the effect of adding detergent to the columns. After this activity, present the associated Density & Miscibility lesson for a discussion about why the column layers do not mix.

'Tricks' tap into children's natural curiosity and can provide the motivation for exploring the underlying mathematics in order to unpick how they are done. This Nrich problem explores a "trick" to provide an engaging context in which to explore place value and in particular 'adding nine' as 'adding ten and subtracting one'.

In this YouCubed task. students examine what happens when you keep continuing a pattern, forever. Students delve into the infinite and find the patterns and their area.

This short video and interactive assessment activity is designed to teach fifth graders about dividing fractions by fractions.

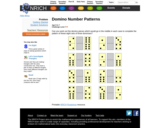
This Nrich problem uses the idea of sequences in a very tangible form. Children will need to recognize odd and even numbers as well as be able to count fluently both backwards and forwards. They will also have opportunities to justify their answers.

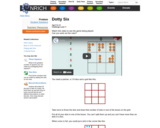
The Nrich game as introduced is intended for children who are just beginning to become confident with small numbers. However there are many variations, some suggested below, that make it suitable for older children. As with many of the NRICH games, consolidation of basic number facts is combined with an element of strategic thinking.

How many calories are in your favorite foods? How much exercise would you have to do to burn off these calories? What is the relationship between calories and weight? Explore these issues by choosing diet and exercise and keeping an eye on your weight.

Unit fractions are the first fractions children meet, and here we discover some very surprising and interesting characteristics of these familiar numbers. Some of these characteristics were known to the ancient Egyptians whilst other conjectures are yet to be proved. Whilst meeting both old and new mathematical ideas, students can improve their fluency in addition and subtraction of fractions and be challenged to generalize and explain their findings.

This lesson unit is intended to help you assess how well students are able to: solve simple problems involving ratio and direct proportion; choose an appropriate sampling method; and collect discrete data and record them using a frequency table.